workshop 2: lets grow up a bit more~

1·         For the second workshop, I have broaden a little bit my "mathematical horizon" as I explored on subitising, counting, number sequences, addition/subtraction, multiplication, number facts strategies and mental computation. So, I would explain on some of the strategies that adults particularly teachers can practice in order to support the development of these skills which are important for students in order to move to the next stage in growing up with math.
   
      1. Subitising

Children acquire sight recognition of a quantity in subtising where they can automatically know the numbers of objects when they see them (Clements, 1999). Children may develop this ability through mathematical knowledge such as the domino pattern or without them (Clements, 1999). In classroom, teachers can use subitising flash cards (picture below)to teach this skill where numbers are represented via the dots on the cards.

Besides, the koala race game is also suitable to teach children subitising where they can play in groups. Each student will have 1 koala as a counter, then, they will take turn in throwing the dice and the counter can move forward if their chosen number appears on the dice. The winner is the first person who reaches the final.

The fly swatter game is also challenging and enjoyable where it is also played in groups. One person will place the cards and the others will have a fly swatter each person. They have to snap numbered cards that appear twice. Thus, children’s automaticity in subitising can be enhanced through this activity.

·         








       2. Counting

There are many ways to teach children how to count. Teachers or parent can use story books which are interested for the children. In illustration,  My big book: Winnie the Pooh is suitable for children to learn counting through shared reading whereby the characters introduce the numbers in a sequence and there are also colourful pictures which appeal to them. Teachers can ask guiding questions such as:

• what number do you think come after number 2?
• can you count how many bags of carrot rabbit has in this story? 

These questions will be able to support students to count in meaningful context.

Additionally, technology is also a great choice in teaching children counting skills since they love using technology in learning. For example, in babyfirsttv.com, there are many fascinating videos for children in supporting their learning including counting. The picture beside is on counting one until five where there are 5 chicks and children can follow the video in counting the chicks. Children can also play games which are available in this website.

3. Forward number word sequence/number word after/backward number word sequence/number word before

For these skills, teachers can give children big numbered cards and ask them to arrange them on a cloth line using cloth pegs. For example, for number word sequence, they would arrange from 1,2,3,4,5,6 and etc. They can also comprehend the ideas of number word after, before and backward as they move the cards according to teachers instructions and questions such as: 

• where do you think number 5 should be?, beside which number?
• what is the number before number 11?
• lets read aloud the numbers backward starting from number 12 

This activity is also appeal for children as it involve active participation and movement in classroom. Teachers can also ask children to hold the cards and arrange themselves in order to enhance these skills.

·        4. Early addition/subtraction

Children can practice these skills using concrete objects before they can do addition and subtraction abstractly in their mind (Smith, 2009). Teachers can use many concrete objects which are usually used for counting such as sticks, pebbles or beads. For instance, tens frames can be used to teach addition and subtraction as children can build a clear picture in their mind for computation before they can move on to mental computation. 

addition

Eg: 3 + 2=

Children can use different coloured counters in order to understand the addition. So, 2 greens plus 3 purples equal to 5. The answer is 5

subtraction

Eg: 5 - 2 =

1.

There are 5 koalas on the coach, 3 reds and 2 greens.

2.

Take away 2 green koalas

3.

  So, there are 3 red koalas left on the coach now.

It is easier for children if teachers associate colours in teaching addition and subtraction since they can easily see the idea of 'adding to' or 'take away'.

·         5. Early multiplication/division
       multiplication- teachers can show the multiplication table for children to show that multiplication is by adding sets of equal numbers;for example the table below which explains that 2 multiply by 2  is 2 smiles + 2 smiles.so, the answer is 4. Besides, by using pictures to explain multiplications, children would understand the concept better.

      division- teachers can use the idea of sorting; for example, 6 ÷ 2=, teachers can explain using the stars in picture below that 6 stars divided equally in 2 based on their colours would produce  3 groups of stars. The different colours can help children in comprehending that the stars has been divided equally according to their colours.

     Teachers can also let children play this fun leap froggy division game as they become better in division. The game can be played in groups where each person would take turn in throwing two dices. For instance, the number on the dices are 4 and 6, so, the person can choose the number 46 or 64. Then this number need to be divided by 6 which the full number of a dice. For example, he/she chose 46, so, 46 ÷ 6 = 7 and the balance is 4. Then he/she can move the counter to number 4.  The winner is the first person who reaches the final.This game is very exciting and can improve children division skill.

      6. Number facts strategies for either addition and subtraction or multiplication and division

addition

6 + 9 = ?


6 red dots and 9 black dots.

one of the red dots will fill in the tens frame and the answer is 15. 

children could clearly see this process as the card is folded under to show the changes of red dot.

multiplication

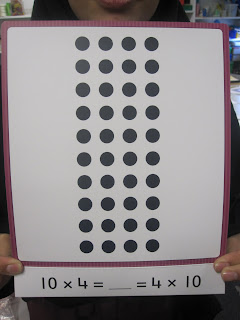
This card can show to children that 10 × 4 is the same with 4 × 10 which equal to 40.

Then, the card can be folded upward to hide the 10th row and show that 9 × 4 is the same with 4 × 9 . The answer is 36.


















7. Mental computation strategies

Teachers can teach mental computation by using hundreds chart especially when solving bigger numbers. There are two types of hundreds chart which are zero hundreds chart and one hundreds chart. Children can use one counter to practice this skill; for example:


using one hundreds chart, 57 + 24  =


1. Firstly, put the counter on number 57



























2. Then, move two row downward which also means add 20



























3.Finally, move 4 box forward to number 81 which means add another 4. So, the answer is 81

Reflection

When I was in in primary school, I  first learnt computation by using fingers and then using pebbles as counters. In learning addition and subtraction, my teacher used language such as add, minus or take away in order to understand the concepts. Then, I moved to using computation using numeral numbers; in illustration, to solve 3 + 2, we would have the standard pattern of,

       3

+    2

----------

      5

------------

This pattern would be used in learning addition, subtraction as well as multiplication. For division, I usually use 

The most interesting things is that I have just realise that there are many other ways in solving mathematical problems for children, after the second workshop. In my opinion, I was drilled to use these solutions during my primary school that I did not explore on other ways in solving addition, subtraction and etc. Besides, these are the standard answers required when answering exam questions in Malaysia as we need to show the computation for exam. I think teachers should let children to explore various ways in learning computation so that their mind would not be rigid to one way in computing numbers. Thus, in future, I would let my students to have their own creative thinking in solving mathematics in order for them to have a better comprehension on mathematical concepts. it is also due to the fact that mathematic is a 'maze' and I should let them use different path in order to get to their destination.


References:

Clements, D. H. (1999). Subitising: what is it? why teach it? Teaching children mathematics ,   5 (7), 400 - 405. Retrieved from Queensland University of Technology Course Materials.

Milne, A. A., & Shepard, E. H. (2010). My big book: winnie the pooh. Bath: Disney Enterprises Inc.

Smith, S. S. (2009). Early childhood mathematics. Boston: Pearson.

Division [ Image]. Retrieved August 29, 2011, from                                                        coolmath4kids.com                                               

Workshop 1:Mathemathic and Mina (me!!!)

How do I view Mathematics? 

In one of the tasks given, there was a statement that said, "Doing a math problem is like finding your way through a maze. There are lots of possible pathways to go down". I feel that a Maze is really suitable to describe my view regarding Math because in a maze, even though there are many ways in order to get to the destination, but most of the time I got lost in it. Mathematics for me is a slow process that I always need time to understand them. Besides, growing up as a mild Asperger person, a maze-like feelings towards math is not a surprise since some Aspergers have problems in mathematics (Griswold, Barnhill, Myles, Hagiwara, & Simpson,  2002 )

What is my assumptions about early childhood mathematics?

My assumptions on early childhood mathematics is that children would learn them when they enter kindergarten at the age of 5 as they would have a formal learning on numbers and some addition. However, after the first workshop, I realize that children grow up with mathematics as they explore the things around them. Likewise, i was once a child who may not know what is 1-10 but in truth, I had already experienced mathematics while I looked at the different flowers at my backyard, played with my mothers’ shoes, when we baked some cookies or looked at the heights of the coconut trees around my house. That was mathematics!! Thus, I should not say that i’m not good in mathematics as I am used to the concepts of mathematics since I was a small child.

Now, I am a student  and I also work part timely as a babysitter where I would take care of kids from different age groups. Therefore, studying this subject has made me more conscious about the kids that I have babysitted. Some beginning processes started to make sense for me and I also reflect on my own development in mathematics when I grew up.

The beginning processes in mathematics are:

1. Identifying and describing attributes
2. Matching
 3. Sorting
4. Comparing
 5. Ordering
 6. Patterning

These processes are the vital before moving on to higher mathematical concepts. Children use their 5 senses in these processes which are, taste, see, smell, hear and taste (Irons, 1999). Besides, it is also essential to highlight Vygotsky theory of zone of proximal development which is the gap between what children can do by themselves and with assistance from adults (Smith, 2009,p. 10). Thus, they need the adults and their peers in order to understand the beginning process through negotiation with friends and scaffold by adult (Smith, 2009,p. 10).

1. Identifying and describing attributes

Children learn how to be more perceptive in this process as they talk about similarities and differences of two objects. Language is central in identifying and describing in details of the objects because they have to make reasoning of the similarities or distinction made. For example, when they look at the two boxes in the picture above, they would say that they are the same because both are gift boxes or have the same shape. They would also make reason of why these two boxes are different from each other based on their colour, decoration and even the texture of the box for instance the pink box is smooth and the yellow box has uneven surface.

2. Matching

Matching on the other hand allows children to choose and describe two objects that have similarities with each other (Irons, 1999). Likewise they would choose on the attributes that they think the two objects share for example; when given the miniature dinosaurs above, let the children match them together and then ask them to talk about their choice. They may talk about how the dinosaurs in one group are the same because both of them have long tails or have sharp claws. Further, they can move to matching activities which involve symbols or illustrations such as matching pictures of dinosaurs with the outlines of dinosaurs (Irons, 1999).

3. Sorting

When given large number of objects, children can do sorting where they would match them according to more than one attributes and group them together (Irons, 1999). Adult can use the wooden 3 dimensional objects and let children sort them; additionally, questions for scaffoldings purpose can be asked such as, 'how do want you arrange the objects?' 'Can you explain the features of each group that you have sorted?'. Besides, children can also try sorting real things for example, the fruits in the picture above.  

Children also can do sorting using this board game where 2 dimensional pictures are used; this game particularly is highly challenging for children and suitable for group activities since they can interact with peers in making decisions to complete the board.

4. Comparing

In comparing, children learn how to observe the differences in various characteristics such as size, weight, temperature or loudness (Smith, 2009,p. 57).  They can compare weight by lifting up two objects and decide which one is heavier. For instance, they can be given two luggages and adult can ask them questions such as 'which one is bigger than the other?' or 'which one do you think is heavier than the other', then, allow them to measure the size using measuring tape and decide the weight by lifting each luggage. Comparing process promotes children to practice using comparing words such for example, ‘smaller’ or ‘bigger’ than the other objects; nevertheless,adult need to model their usage to children in order to enhance children understanding in this process.

5. Ordering

Children learn to organise three or more objects or pictures in decreasing or increasing manner (Irons, 1999). For example, adult can give a set of Russian dolls and ask them to arrange them according to height; children can be scaffolded via comparing questions such as 'which one do you think is the tallest?' and 'which one do you think should be the first when you arrange the dolls ?'. Other than that, they can also practice ordering using ‘realia’ namely, everyday objects such as books in different sizes.

7. Patterning

Children make easily identified and expected repetition of objects or pictures (Irons, 1999). For example, children can make a pattern using the jigsaw mat above by ordering a repetition of the colour yellow, red and blue. In addition, they can make a pattern using colourful shapes or beads.

Learning experience during math class

Patterning- I always thought that pattern can only be done using objects but the first workshop had made me realize that it can also be done in the form of sounds as long as it is done in a pattern either ascending  or descending. watch the video below to understand more on making a sound pattern~^^

These bells were used for making the sound pattern, in classroom, teachers can ask children to make their own sound pattern by shaking it once,  twice and then three times repeatedly. It is a fun activity especially for children who learn best through musical instruments (Armstrong, 2009)

Besides, teachers can go further in teaching pattern by using keyboard where the notes do, re, mi, fa so, la, and ti are usually used in pattern to form music. Labelling the key on the board would help as scaffold for the children. I also believe that these type of activity would make children aware that mathematical concepts are everywhere around them.

Learning experience during babysitting a soon to be 4 years old kid~

This is Talah, i’ve babysitted her since last year. So, as mentioned before, taking this subject had made me aware of her mathematical development as well as her language development. One significant event which made me realize that she is doing the beginning process is when we were playing Lego together as she sorted the Lego according to the colours when she put them back in the container. It was so amazing to see her putting the yellows and then blues before the greens into the container. Besides, when I asked her which Lego tower is taller between the two towers that we have built earlier, she pointed out at the correct tower. Therefore, this had made reflect that I must have gone through the same process when growing up. In future, I would be more observant towards the kids that I babysit in order to help them develop their mathematical skills and at the same time reflect on my teaching abilities.

references:

Armstrong, T. (2009). Multiple intelligences in the classroom. USA: ASCD

Griswold, D. E., Barnhill, G. P., Myles, B. S., Hagiwara, T., & Simpson, R. L. (2002). Asperger syndrome and academic achievement. Focus on Autism and Other Developmental Disabilities. 17(2), 94-102.

Irons, R. (1999). Numeracy in early childhood. Educating Young Children: Learning and Teaching in Early Childhood, 5(3), 26-32.

Smith, S. S. (2009). Early childhood mathematics. USA: Pearson education

Grist, M. J. Story craft [image]. Retrieved August 23, 2011 from

http://www.michaeljohngrist.com/2010/08/writing-blog-6-a-thesaurus-for-the-maze/

m new

hye im new... 

so excited!

lets cruise along with me(n my dear Talah) in my mathematical journey